
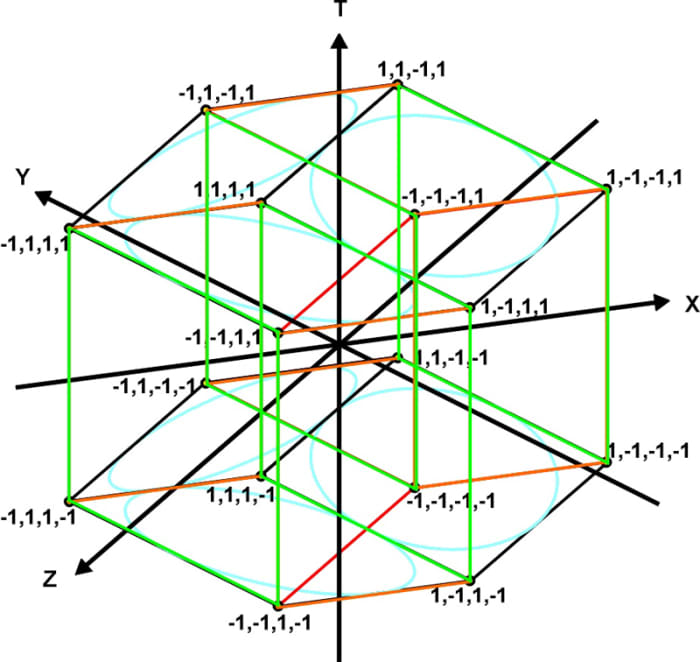
claimed that a STDS is a -diagnosable system provided that the system does not have a node such that its neighbors are all faulty simultaneously, the probability of which is very low. proposed a concept called a strongly -diagnosable system (STDS). The ratio implies that the probability that there exists some node such that its neighbors are all faulty is very low. However, the number of node subsets such that its size is and it incorporates all neighbors of some node is at most. For example, consider an -dimensional hypercube network the number of node subsets such that its cardinality is is. In practice, the probability that all neighbors of a node are synchronously faulty is very low. In other words, in the PMC model, the diagnosability of a system with missing edges depends on the upper limit of the minimum degree of. In the PMC model, when we consider the diagnosability of a system with missing edges, if all neighbors of some node are faulty, then it is impossible to judge whether or not the node is fault-free. Since then, results corresponding to the PMC model have been widely reported (see ). put forward the concept of a one-step -diagnosable system and of a sequentially -diagnosable system. “PMC” stands for Preparata, Metze, and Chien, as this model was first proposed by Preparata et al. The fault diagnosis model in this paper is the PMC model. In system-level fault diagnosis, two models that are typically used as fault diagnosis models are the PMC model and the comparison model. We call this edge the crossing edge between and. Then there must be another node satisfying. can be decomposed in two -dimensional hypercube networks and along the th-dimensional edges. For two nodes and in, if and are connected by a link and their addresses differ in the th position, then this edge is called the th-dimensional edge. If the address of node and the address of node are different in exactly one bit position, then they are connected by a link, and vice versa. A binary -bit string, e.g.,, can be employed to denote the address of a node in. An -dimensional hypercube network, denoted as, consists of nodes and edges. The hypercube network structure is a popular topology in modeling a multiprocessor system. The diagnosability, a key measurement of self-diagnosis capacity, of a system is the upper limit on the number of faulty processors that a system is certain to identify. The process of identifying faulty processors is referred to as fault diagnosis, which has been widely studied in. Whenever a faulty node is identified by the system, it should be repaired or replaced with an additional one. Hence, in the system design, the problem of self-diagnosis should be considered. Faulty processors in a multiprocessor system will affect the reliability of the system.
In other words, there may exist faulty processors in such a system. In a multiprocessor system incorporating a large number of processors (nodes), some processors may fail. If the RD of each node is greater than, then the SLD of each node is also equal to its RD, no matter how many faulty edges exist in. Finally, we discuss the SLD of a node for an incomplete hypercube network and obtain the following results: if the minimum RD of nodes in an incomplete hypercube network of -dimensions is greater than, then the SLD of any node is still equal to its RD provided that the number of faulty edges does not exceed. We determine that the SLD of a node is equal to its remaining degree (RD) in an incomplete hypercube network, which is true provided that the number of faulty edges in this hypercube network does not exceed. Moreover, we explore the SLD of a node of an incomplete hypercube network. Based on these results, we conclude that in a hypercube network of dimensions, denoted by, the SLD of a node is equal to its degree when. In addition, a few important results related to the SLD of a node of a system are presented. In this article, a new concept regarding diagnosability, called strong local diagnosability (SLD), which describes the local status of the strong diagnosability (SD) of a system, is presented. In the research on the reliability of a connection network, diagnosability is an important problem that should be considered.


 0 kommentar(er)
0 kommentar(er)
